In a recent article,1 Alberto Moniz contributed a useful discourse on the reasons why covalent and ionic compounds do or don’t form. From my experience, it assists some students to understand concepts if they can see concrete numbers. For this reason, I thought it worthwhile to provide examples to illustrate why certain compounds exist and others do not. The arguments will be based on energy considerations. Though it is the net free energy change which is the ultimate determining factor, relating existence of a compound to the exothermicity of the formation process from the elements (or more correctly, enthalpy change) works well most of the time.
The (Non-)existence of covalent compounds
Which compounds form and which do not form, is an interesting field in itself. Dasent wrote a whole book on nonexistent compounds,2 while Appelman described the difficulties of synthesizing perbromates and hypofluorous acid.3
The classic case was the discovery of noble gas compounds.4 Noble gas compounds are now back in the news. The Earth’s atmosphere should contain much more xenon than is, in fact, the case. Recently, xenon dioxide has been synthesized, and it is proposed that the “missing” xenon is trapped as XeO2 units within the SiO2 units of quartz and silicates deep within the continental crust.5 Thus it seems appropriate to choose a noble-gas-compound example. Here, I illustrate why covalently-bonded xenon tetrafluoride exists while xenon tetrachloride almost certainly will never be synthesized.
The possible formation of a covalent compound from its elements can be considered in terms of bonds in the elements being broken and bonds in the compound being formed. A process that is significantly exothermic overall indicates a significant possibility that the compound could exist.
For the formation of xenon tetrafluoride, the overall chemical equation can be written as
Xe(g) + 2 F2(g) → XeF4(s)
The process can be considered as occurring in a series of theoretical steps
- The endothermic breaking of two moles of fluorine-fluorine covalent bonds.
- The exothermic forming of four moles of xenon-fluorine bonds.
- The small exothermic deposition of solid xenon tetrafluoride.
The energy cycle with the numerical values is shown (Fig. 1).
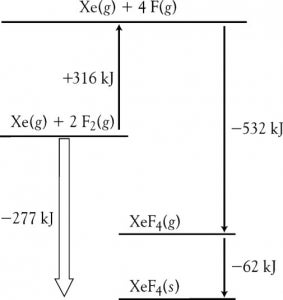 Fig. 1. The theoretical energy of formation diagram for xenon tetrafluoride (from ref. 6)
Fig. 1. The theoretical energy of formation diagram for xenon tetrafluoride (from ref. 6)
Thus the process for the formation of xenon tetrafluoride is significantly exothermic and XeF4 would be postulated to exist. In fact, the compound was first synthesized in 1962.
From a thermochemistry perspective, xenon tetrafluoride exists because the two fluorine-fluorine bonds to be broken are quite weak (F−F = 158 kJ∙mol−1) while there are four xenon-fluorine bonds to be formed — even though each of these is even weaker (Xe−F = 133 kJ∙mol−1).
So why would the synthesis of xenon tetrachloride be extremely unlikely? The chlorine-chlorine bond (Cl−Cl = 240 kJ∙mol−1) is significantly stronger than the fluorine-fluorine bond. In a previous article, I showed that a theoretical value for the xenon-chlorine bond is less than that of the xenon-fluorine bond (Xe−Cl = 103 kJ∙mol−1).7 So, a similar energy cycle for gaseous xenon tetrachloride would give a net energy change of +68 kJ∙mol−1). Even if a solid were formed, the cycle would not involve a significant degree of exothermicity. Thus one can say reasonably confidently that mixing xenon gas and chlorine gas would not lead to the formation of xenon tetrachloride.
The (Non-)-existence of ionic compounds
For ionic compounds, an ion-formation pathway must be employed. We can illustrate the concept using the formation of sodium chloride.
[mathjax]Na(s) + Cl2(g) NaCl(s)
The process in this case can be considered as occurring in the following theoretical steps:
- The vaporization of one mole of sodium atoms.
- The breaking of one-half mole of chlorine molecules.
- The first ionization energy of one mole of sodium atoms.
- The electron affinity of one mole of chlorine atoms.
- The deposition of one mole of sodium ions and one mole of chloride ions to form the crystal lattice.
The energy cycle with the numerical values is shown below:
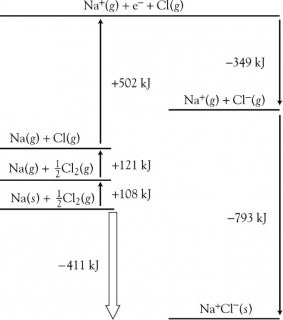
Fig. 2. The theoretical energy of formation diagram for sodium chloride (from ref. 6)
It can be seen from this figure that the two major factors in the energy balance are the ionization energy necessary to form the cation and the energy released from the formation of the crystal lattice. We can use this fact to see why, for example, magnesium and fluorine form MgF2 and not MgF nor MgF3. As can be seen from Fig. 3 (next column), the formation of MgF would theoretically be somewhat feasible. However, the energy required to remove magnesium’s other valence electron is more than compensated by the stronger crystal lattice formed by packing the higher-charge Mg2+ ions with the F− ions. So the formation of MgF2 is energetically preferred.
So why not go one further and ionize a third electron from magnesium? For the lattice energy would be much higher still.
It is certainly true that the lattice energy would be about double of that for MgF2. However, the third ionized electron has to come from an inner shell and requires an enormous quantity of energy for the ionization process. MgF3 can never be formed. This is also shown in Fig. 3.
Ionic or covalent bonding?
Though the impossibility of the formation of MgF3 seems energetically beyond doubt, one must be careful when one says “never”. After all, we thought we “knew” that mercury could only form ions with a +1 and +2 charge.
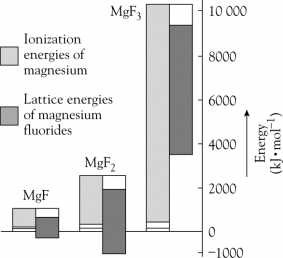 Fig. 3 A comparison of the energies of formation of MgF, MgF2, and MgF3 (from ref. 6)
Fig. 3 A comparison of the energies of formation of MgF, MgF2, and MgF3 (from ref. 6)
Now we know that it is feasible to synthesize HgF4, in which mercury has an assigned charge of +4.8
Hg(l) + 2 F2(g) → HgF4(s)
As it happens, for this “borderline metal” the compound is not ionic at all, but covalent. In fact, with an Hg−F bond energy of about 180 kJ∙mol−1, with hindsight, the formation of this molecule should be quite expected upon energy grounds:
Energy change =
(breaking of 2 mol of F−F bonds) – (formation of 4 mol of Hg−F bonds)
= (316 kJ) – (720 kJ) = −404 kJ∙mol−1
References
- A. Moniz, “Constructing meaning from the octet rule,” Chem 13 News, October 2011, page 4.
- W.E, Dasent, Nonexistent Compounds, Edward Arnold, 1965.
- E.H. Appelman, “Nonexistent compounds: Two case histories,” Accounts of Chemical Research, 6, 1973, pages 113-117.
- P. Laszlo and G.J. Schrobilgen, “One or several pioneers? The discovery of noble-gas compounds,” Angewandte Chemie (International Edition),1988, 27, page 479.
- D.S. Brock and G.J. Schrobilgen, “Synthesis of the missing oxide of xenon, XeO2, and its implications for Earth’s missing xenon,” Journal of the American Chemical Society, April 2011, 133 (16), pages 6265-9.
- G. Rayner-Canham and T. Overton, Descriptive Inorganic Chemistry, 5th edition, W.H. Freeman, New York, 2010.
- G. Rayner-Canham, “The noble gases and their relevance to 21st century chemistry teaching,” Chem 13 News, November 2006,
page 4. - “Wikipedia, Mercury(IV) fluoride”






