I study the turbulent fluid dynamics of the atmosphere at large and small scales. Atmospheric turbulence is strongly influenced by buoyancy, the rotation of the Earth, and phase changes of water. Numerical simulation plays a central role in my research. By numerically integrating the equations of motion for the atmosphere, we can perform virtual experiments of different flows. Such experiments allow us to test our theoretical understanding and generate new insight. I use a variety of different numerical tools, including spectral methods for idealized turbulence simulations, and mesoscale models (such as WRF) for more realistic configurations.
Stratified Turbulence
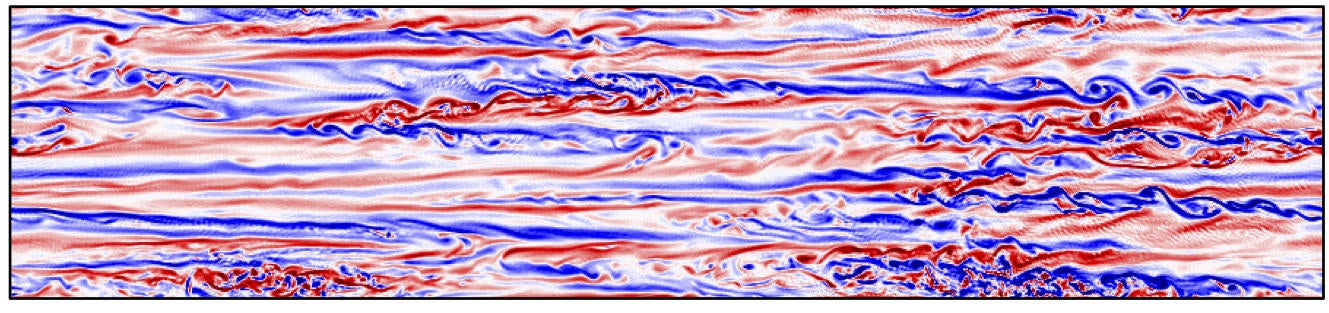
A fluid with uniform density stratification provides a clean, idealized framework for the study of vortices and waves in atmospheric turbulence. Strong density stratification leads to a layered flow structure of "pancake" vortices. If the Reynolds number is large enough, these layers develop shear instabilities that ultimately transition to more isotropic three-dimensional turbulence at smaller scales. The figure below shows a vertical slice of horizontal vorticity from a simulation with strong stratification. Layers, instabilities, and small-scale turbulence are all clearly visible. The physics of the energy cascade in both homogeneous and wall-bounded stratified turbulence are areas of ongoing research.
Mesoscale and Rotating Stratified Turbulence
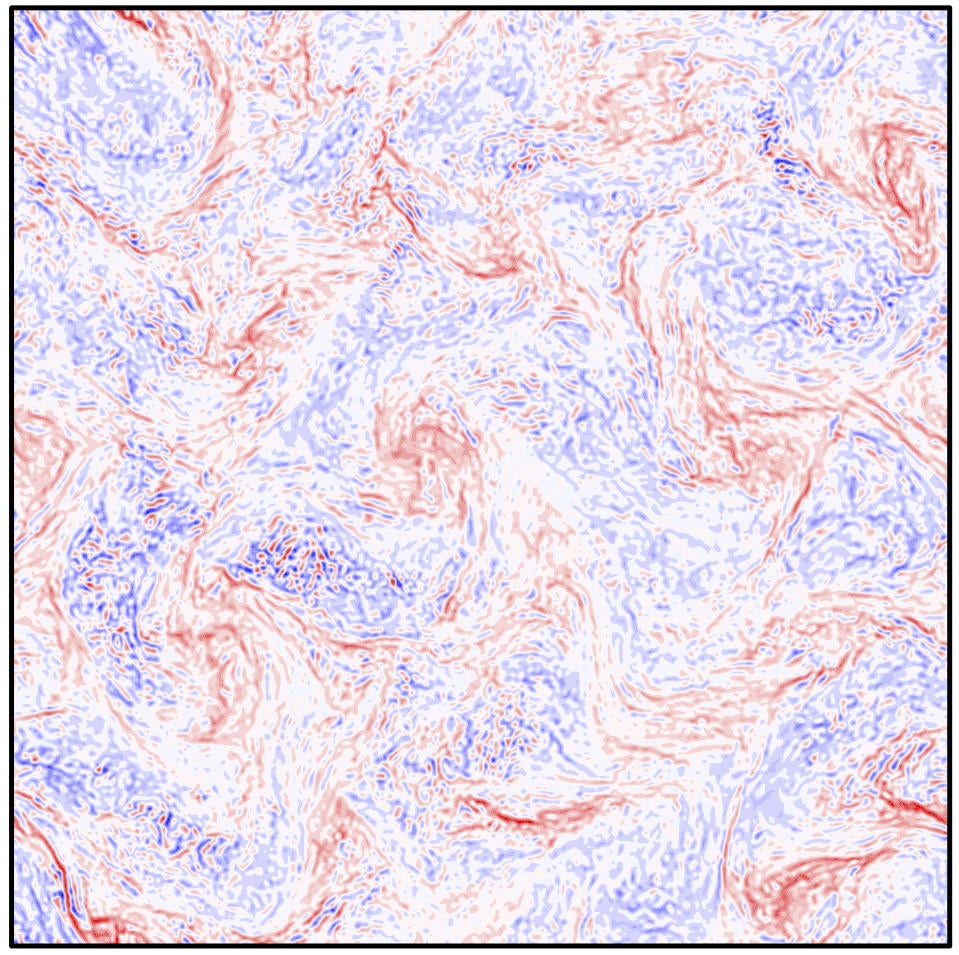
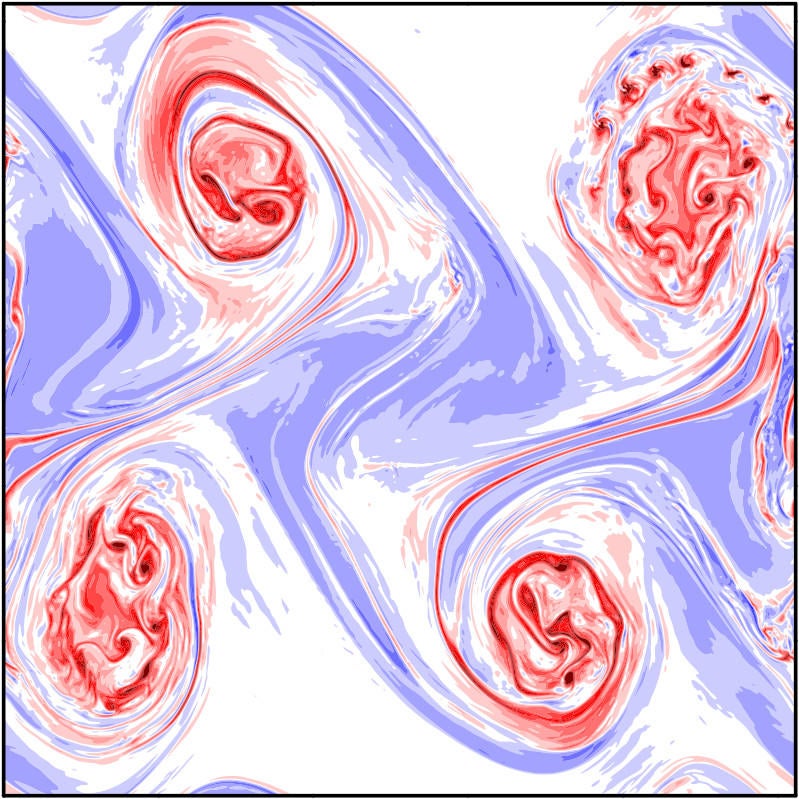
The atmospheric mesoscale comprises a rich array of dynamics including fronts, clouds, tropopause folds, gravity waves, and turbulence. These features conspire to yield a kinetic energy spectrum with an approximately -5/3 power spectrum that has puzzled researchers for several decades. A fundamental question is whether this spectrum results from a downscale cascade of energy from synoptic scales, the role of gravity waves and vortices in such a cascade, and the corresponding role of moist processes in directly energizing the mesoscale. My research employs high-resolution simulations of dry and moist mesoscale turbulence to investigate this question. The figures below shows horizontal slices of relative vorticity in idealized rotating-stratified turbulence (left) and dry baroclinic instability (right, by Master's student Matthew Ambacher). In both cases, the cascade to small scales is apparent.
Large eddy simulation
Ideally, numerical simulations of tu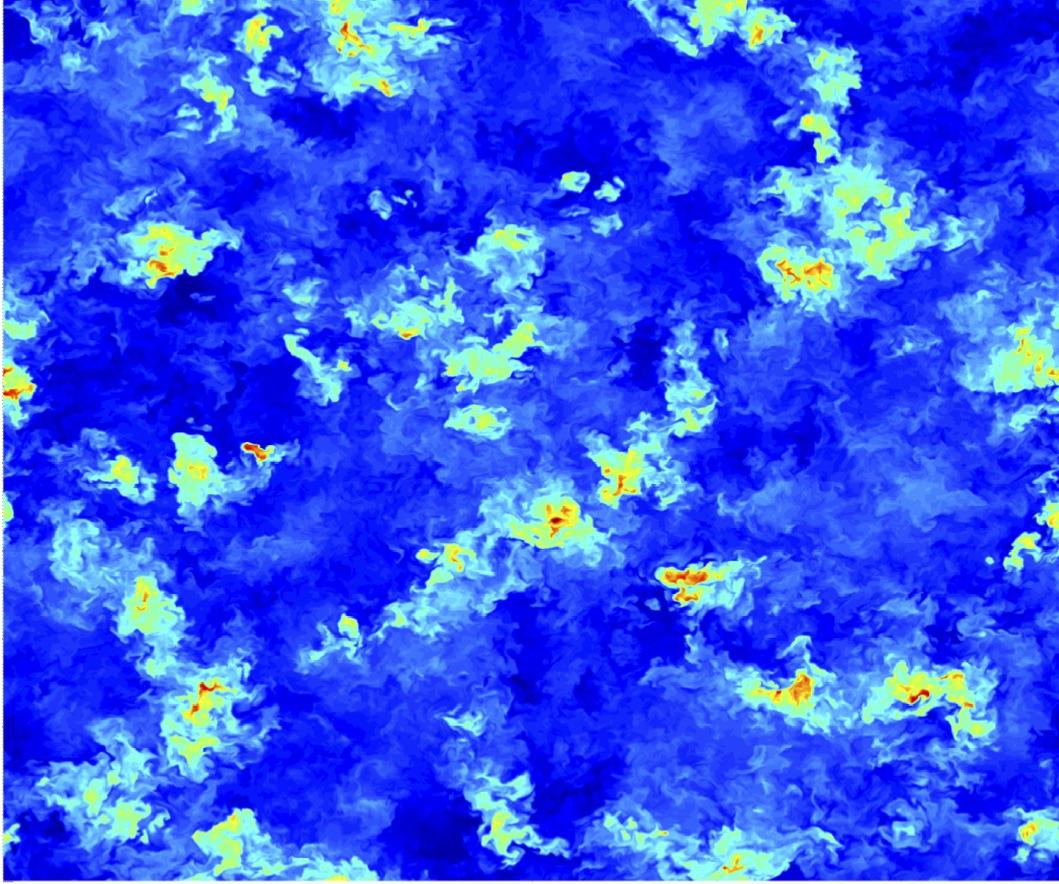
rbulence use a grid spacing that is fine enough to resolve eddies of all length scales. This approach is called direct numerical simulation (DNS). In the atmosphere, the smallest-scale eddies are around 1 mm in size, while the largest scales may be 1, 10, 100, or even 1000 km in size. As a result, even with the largest supercomputers, it is usually impossible to perform DNS for realistic atmospheric flows.
Large eddy simulation (LES) is an alternative approach for turbulence simulations. In LES, only the large-scale turbulent eddies are explicitly resolved, while the effects of smaller-scale eddies are parameterized. The figure on the right shows a vertical slice of temperature from LES of a convective boundary layer. The grid spacing is 5 m, which is more than 1000 times larger than the smallest-scale turbulent eddies. This simulation was performed by Master's student James Sandham (see animations here). My research on LES investigates the effects of density stratification on sub-grid turbulence parameterizations.
